Domingo Andria

MPhil Student in System Dynamics, University of Bergen, Norway - domingoa@ifi.uib.no

Doctoral Program Student, University of Palermo, Italy - andria@unipa.it - ricopa@unipa.it

Abstract: The purpose of this paper is to demonstrate how a S.D. model could support financial decision makers in understanding processes related to the management of the firm's liquidity position. A causal dynamic simulation model will be constructed in order to depict feedback loops generated by the so called float, i.e. the delay between the time when a cheque is issued by a company and the time when it is cleared. There are two kinds of float: the payment float and the availability float. The first one occurs when a firm pays its suppliers by cheque; the second one occurs when a firm receives a cheque from one of its customers. Managing floats may be of a great importance for a firm, both on a financial and on an economic point of view. In fact, owing to delays generated by floats, a company may benefit from extra funds, free of interest. Consequently, other conditions being equal, levering on float it will be possible to increase investments and - if operating unit revenues will be higher than costs - to achieve a growing internal flow of funds that will allow to support growth. Traditional reporting fails to depict such phenomenon. Dynamic modelling and simulation could support financial managers to understand how to lever on floats in order to raise higher funds from daily transactions to support their current financial needs.

Problem Description

Companies do not keep their cash in a little tin box inside the company, but they keep it in a bank deposit. This means that the same company is characterised by having two different financial ledgers (or balances): the cash balance and the bank balance.

Float management plays its role only in this difference between the two balances referring to the same company.

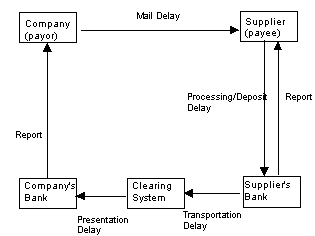
To understand how float intervenes in this difference we need to know what happens when companies withdraw money from their account or pay money into them. In particular, we need to understand by which components the "cheque system" is affected, and how a cheque is used to transfer value from the company (payor) to one of its supplier (payee). Figure 1 resumes this particular process. As result of the delayed process, the company's bank will not learn anything about the cheque issued by the company until it has been received by the supplier, deposited at his bank, and finally presented, through the clearing system, to the company's bank for payment.

Fig.1: The "cheque's subsystem diagram"

During this time the company's bank continues to show in its ledger that the company has the same balance that it had before issuing the cheque. While the cheque is being cleared, the company obtains the benefit of an extra sum (the value shown in the cheque) in its bank balance. The difference between the cash recorded as deposit on the company's book and the increase in the available balance at the bank is defined as "float". In the cash management business there are several kinds of delays, and so people refer also

to several kinds of float. Figure 2 summarises the three sources of float:

the time that it takes to mail a cheque; the time that it takes the supplier to process the cheque after it has been received; the time that it takes the bank to clear the cheque and adjust the company's account. Of course the delays that help the payer hurt the recipient. Recipients, speeding up collections try to reduce delays to get available cash sooner. Payers, slowing down disbursement, prefer delays to be able to use their cash longer.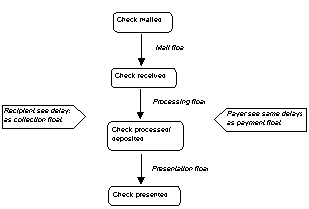

Fig.2: Different kinds of float

The System Dynamics Approach

We propose to describe and to study the phenomenon above with a dynamic simulation model and test our assumptions by validating our model and apply our model to support financial decision makers in understanding processes related to the management of the firm's liquidity position. We have applied the system dynamics methodology (Richardson & Pugh, 1981; Forrester, 1961) to construct a causal dynamic simulation model. The model has been grounded in existing theories concerning the structures being described and theories of dynamic systems.

In particular, what has been more emphasised in the model, is the role played by the delays in such systems. We have demonstrated how the amplification due to the different kinds of delays in the system, can be used by the financial manager to lever on float to increase investments and - if operating unit revenues are higher than costs - to achieve a growing internal flow of funds that will allow to support growth. Our model has completely, explicitly and transparently described how three interacting subsystems interact over time:

• the company's cash balance sector; it represents the company's financial structure,only considering its cash balance. It is possible to observe the behavior of the money available to the company for the income and the expenses experienced by the company over time.
• the float sector; it reproduces all the process through which float is created. In particular, the three kinds of float mentioned above are represented. The main role is played by the different delays in the cheque process.
• the company's bank balance sector; the effects of float on the company's bank balance are considered here. Initially the effects of float comparing the cash balance available to the company with the bank balance available to the same company are examined. Subsequently, the structure reproducing the company's bank balance is "completed" by the "interests structure" to show how the float's effects and the benefits gained with it are increased by the interests added into the bank account.

Actual Results

Difference between the company's cash balance and the available balance at the bank :

Fig.3: Difference between cash and bank balance

To show the difference between the company's cash balance and the available balance at the bank, are plotted, in the same graph, the two lines representing the two different types of balance: the cash balance and the bank balance. "The bank line"(representing the money available to the company in its deposit account), shows, for a certain time, a value of available

money higher than the money represented by the "cash line" (representing the money available to the company in its cash).

Float's effects in terms of interests:

It is possible to identify two different types of benefit for the company:

• benefit as extra money in the bank deposit;
• benefit as extra money in the interests earned on the bank account;

"Bank balance-1" reproduces the case when the money gained by the company as a consequence of the float created through the cheque process is not considered; on the other hand, "Bank balance-2" reproduces the case when it is taken into consideration. In conclusion, the company, with the extra money obtained because of the delay in the cheque processes , obtains the benefit of extra money as interests, between the day when the bank adds interests and the day when the value corresponding to the cheque is subtracted from its deposit account .

Conclusions 

The causal dynamic simulation model used in our approach has demonstrated that the cash shown in a company ledger is not the same as the available balance in its bank account. The difference is the float. When a company writes a cheque awaiting clearance, the available balance will be larger than the ledger balance. The reason is that it takes time for the company's bank balance to be adjusted downward. During this time the bank balance available at the bank will be larger than the ledger balance available into the company. The financial manager can use this difference. If he can predict how long it takes a cheque to clear, he may be able to play the float and get by on a smaller cash balance. Other conditions being equal, levering on float will be possible to increase investments and - if operating unit revenues will be higher than costs - to achieve a growing internal flow of funds that will allow to support growth.

References

Brealey R.A., Stewart C.Myers, (1991). Principles of Corporate Finance. McGraw-Hill, Inc. - International Edition.

Brealey R.A., Stewart C.Myers, and Alan J.Marcus (1995). Fundamentals of Corporate Finance. McGraw-Hill, Inc. - International Edition.

Coyle R.G. (1996). System Dynamics Modeling - A Practical Approach. Chapman & Hall.

Forrester, Jay W. (1961). Industrial Dynamics. Productivity Press. Cambridge, MA.

Miller D.H. & Stewart C. Myers, (1990). Frontiers of Finance. Basil Blackwell.

Richardson G. P.; Pugh A.L. (1981). System Dynamics Modelling with Dynamo. Productivity Press. Cambridge, MA.

ISDC '97 CD Sponsor